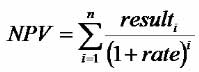The Evolution of Cooperation by Robert Axelrod is an outstanding book. First published in 1984 it has increased in significance with the evolution of the Internet. In the book Axelrod examines how cooperation can emerge and stabilize in multi-participant environments. The book is fascinating as an analysis of the evolution of cooperation, but is of particular interest to anyone seeking to establish effective; social software systems, peer-to-peer networks, or multi-player gaming environments. Axelrod builds his thesis on the analysis of a gaming tournament he organized. He invited multiple people from many different fields; economics, computer science, evolutionary biology, etc, to submit computer programs employing well defined strategies to play a series of games of Prisoner’s Dilemma. Each program played several hundred games against every other program. The results were surprising and enlightening.

The Prisoner’s Dilemma

In the game of Prisoner’s Dilemma there are two players, who each have two choices. Each player chooses simultaneously, to cooperate or to defect. If they both choose to cooperate they both get R – the reward for mutual cooperation. If they both choose to defect they both get P – the punishment for mutual defection. If one cooperates and the other defects then the defector gets T – the temptation, and the cooperative player gets S – the suckers payoff. The dilemma comes from the fact that the best strategy depends on the opponent’s strategy and a smart player knows this, so players must both second guess each other.

The following table shows the scoring system used by Axelrod for the Prisoner’s Dilemma tournament.

	Cooperate	Defect
Cooperate	R=3, R=3 Reward for mutual cooperation	S=0, T=5 Sucker’s payoff, and temptation to defect
Defect	T=5, S=0 Temptation to defect and sucker’s payoff	P=1, P=1 Punishment for mutual defection

The results above are one specific case. Any game is a Prisoner’s Dilemma if it satisfies the following inequalities:

T > R > P > S

And

R > (T+S)/2

(This second inequality means it is better to cooperate than alternately defect and cooperate)

In the Axelrod’s tournament one of the simplest strategies was the clear winner. Axelrod calls this strategy Tit-for-Tat – Cooperate on the first move and thereafter do whatever the opponent did on the previous move. Why this strategy is so successful and what it means is the subject of the rest of the book.

The Shadow of the Future

Axelrod defines a term “w” for weight (or importance) of a future result. He assumes that w always takes a value between zero and one (0 < w < 1). If w is 1 then future results are as important as current results, but if w is 0.5, for example, then future results are half as important as current results. The current value of the next result is calculated by multiplying the payoff by w. High values of w mean the future is more important and low values mean it is less important. The net present value of a series of future results can be calculated according to this formula.

where w is equivalent to 1 / (1+rate)

Axelrod poetically calls the concept of a net present value “The Shadow of the Future”. Increasing w increases the size of the shadow whereas decreasing w decreases the size of the shadow.

The concept of the net present value of future earnings is a common one in economics and is fundamental in many investment decisions. Future earnings are less valuable than current earnings because of risk and opportunity costs. It is not certain that two players will actually meet again or that they will behave in a predictable manner. External influences could change the expected outcome. And there are usually alternative strategies that could be just as rewarding for the same risk.

Collective and Territorial Stability

If the game is played in rounds (where each round consists of many hundreds of turns) and the population of players using a given strategy in the next round is determined by the success of that strategy in the previous round. Then the concept of invasion and collective stability can be examined. A collectively stable strategy is one where a large number of agents using the same strategy cannot be “invaded” by a single agent playing a different strategy. Axelrod shows that some strategies can invade a larger group if there is more than one agent playing the invading strategy. He goes on to prove that Collectively stable strategies are also territorially stable. That is if agents can play only with adjacent agents the same rules apply.

Axelrod’s Propositions

Axelrod defines 8 propositions based on his analysis of the tournament. About half of the book is spent explaining these propositions.

1. If the Discount parameter, w, is sufficiently high, there is no best strategy independent of the strategy used by the other player
2. The Tit-for-Tat strategy is collectively stable if and only if, w is large enough. This critical value of w is a function of the four payoff parameters, T, R, P, and S
3. Any strategy which may be the first to cooperate can vbe collectively stable only when w is sufficiently large
4. For a nice strategy to be collectively stable, it must be provoked by the very first defection of the other player
5. The strategy Always-Defect is always collectively stable
6. The strategies which can invade Always-Defect in a cluster with the smallest value of p (the weighted average score an invader gets from games with other invaders and incumbents) are those which are maximally discriminating, such as Tit-for-Tat
7. If a nice strategy cannot be invaded by a single individual, it cannot be invaded by a cluster of individuals either
8. If a rule is collectively stable, it is territorially stable

How to do well

Axelrod provides four maxims for how to do well as a participant in situations similar to iterated games of Prison Dilemma.

• Don’t be envious – The Prisoner’s Dilemma is not a zero sum game. It is ok if your “opponent” does better than you. In fact if they don’t do at least as well as you then you are not cooperating enough.
• Don’t be the first to defect – Defection is an effective form of punishment but it is costly for both parties, it can lead to long periods of alternating defection. Stay away from defection until forced to act.
• Reciprocate both cooperation and defection – Reciprocity is a double edged sword. You must be prepared to consistently punish defectors as quickly as you reward cooperators.
• Don’t be too clever – Consistency is important, reciprocity works as a strategy if your opponent can predict your next move. Tit-for-Tat is successful partly because it is a transparent strategy.

How to encourage cooperation

Axelrod provides another set of maxims for those trying to encourage cooperation among players.

• Enlarge the shadow of the future – The net present value of future earnings can be increased in several ways. Increase the frequency of interactions between players, and increase the importance of the current game of future games. The importance can be increased by making a players actions a matter of public knowledge. This is the beginnings of reputation.
• Change the payoffs – Changing the payoffs so much that the inequalities defined above no longer hold true will destroy the game, but within the inequalities there is still room for optimization. Increasing R or decreasing T and P can all have encouraging effects
• Teach people to care about each other – Ethics and ritual often evolve in environments of iterative Prisoner’s Dilemma. These emergent features serve to enforce socially acceptable rules of conduct and can change players perception of the game to the point where cooperation is more “desirable” than the temptation to defect.
• Teach Reciprocity – Tit-for-Tat is fair but it has additional advantages. Tit-for-Tat encourages other players to use similar strategies. Players using Tit-for-Tat police their opponents and therefore provide a secondary benefit to other players using Tit-for-Tat
• Improve recognition abilities – Cooperation works effectively where players can recognize each other and remember how a given player behaved last time they met. Recognition and memory can be improved in several ways. Labels stereotypes, status and reputation can all play a part. Labeling players and categorizing them into stereotypes can help make large numbers of players manageable and make it easier to make fast decisions. Reputation is an emergent property of social groups. Players are generally recognized by the group as being reliable or unreliable. This is both a form of labeling and of collective memory.

Shirky’s Restatement

In his recent essay A Group is its own Worst Enemy Clay Shirky simplifies and restates similar findings by suggesting “four things to design for” when designing a social software system:

1. If you were going to build a piece of social software to support large and long-lived groups, what would you design for? The first thing you would design for is handles the user can invest in.
2. You have to design a way for there to be members in good standing. Have to design some way in which good works get recognized. The minimal way is, posts appear with identity. You can do more sophisticated things like having formal karma or “member since.”
3. You need barriers to participation. This is one of the things that killed Usenet. You have to have some cost to either join or participate, if not at the lowest level, then at higher levels. There needs to be some kind of segmentation of capabilities.
4. You have to find a way to spare the group from scale. Scale alone kills conversations, because conversations require dense two-way conversations. In conversational contexts, Metcalfe’s law is a drag. The fact that the amount of two-way connections you have to support goes up with the square of the users means that the density of conversation falls off very fast as the system scales even a little bit. You have to have some way to let users hang onto the less is more pattern, in order to keep associated with one another.

Emergence of Social Structures at the boundary of Cooperation and Defection

These lessons can be usefully applied to a variety of networked communities such as; peer-to-peer networks, multi-player gaming environments, and other social software systems if the community in question is playing a close analogue of the Prisoners Dilemma. This is true when the payoff scheme has equivalents to the payoffs R,P,T,S, and these equivalents satisfy, or can be made to satisfy, the inequalities T > R > P > S and R > (T+S)/2.

In his fine book Complexity: The Emerging Science at the Edge of Order and Chaos. , Mitchell M. Waldrop states that systems evolve most rapidly when they are pushed to the edge of chaos and order because this is the region where complexity emerges spontaneously. The inequalities that define the Prisoner’s Dilemma describe a similar boundary, between cooperation and defection, where complex social structures like ethics, ritual, and reputation emerge. I believe the most interesting social environments are the most adaptive environments, that support the emergence of complex social structures, that are only possible because both cooperation and defection are permitted. I believe it is therefore worth pushing networked environments towards this edge by making cooperation only slightly more attractive than defection. In other words R > (T+S)/2, but only just!

In many cases existing environments could be greatly improved if the payoff scheme were brought more into balance. It is common for the payoff scheme of these environments to be out of balance, either defectors go unpunished or there is no opportunity to defect and everyone becomes a sucker ripe for exploitation. By applying some of the maxims defined by Axelrod the payoff schemes of these environments can be moved towards a more balanced state typical of the Prisoner’s Dilemma. The important point to realize is that the option to defect is necessary because it is this option that drives the emergence of many social structures whose purpose is to encourage cooperation. These emergent features are only found where they are necessary to tip the balance between cooperation and defection in favor of cooperation.

I recently had to setup a home office. The hardest decision to make was which desk to buy. Given that I will be sitting at this desk for many hours and I have lower back problems, from playing too much rugby, I wanted an adjustable desk to match the Aeron chair I already have. It’s surprising that while there are plenty of adjustable chairs around there are not that many adjustable desks. In the end it came down to a choice between the AdjustaBench from Anthro and the Personal Desk from Biomorph. The Biomorph won on price and looks. It came flat-packed in a huge wooden crate, construction took an hour or so and the engineering quality was evident from the precision with which the parts snapped into place. The best features are:

• The ergonomic shape – The split desk allows the back surface to be raised higher that the front surface and is robust enough to support the heaviest of monitors. The curve of the desk matches my arms-reach so everything on the back surface is within my grasp.
• The height adjustment – With a few effortless turns of the hand-crank the surfaces of the desk can be independently raised or lowered. I can even work standing up.
• The cable management systems – All the cables and transformers for charging the; cellphone, pda and laptop are held on a tray under the desk surface out of sight.
• The robust engineering – Living San Francisco means one is always looking for a place to hide during The Big One. I now know where I shall be.

In 1946 between 8th July and 31st August the Moore School of Electrical Engineering at the University of Pennsylvania held a special course entitled Theory and Techniques for Design of Electronic Digital Computers. The course was organized in response to interest generated by; the schools public announcement of the ENIAC, and the publication of The First Draft of a Report on the EDVAC. 1945 by Jon von Neumann. Attendance was by invitation only and the “Students” were selected from the leading experts at the major institutions working on the development of computing devices in the US and UK. At the time of this event there were only three published designs for a stored program computer and it was expected that all those present were familiar with these documents.

• The First Draft of a Report on the EDVAC by Jon von Neumann. 1945
• Proposed Electronic Calculator.by Alan Turing. 1945.
• Preliminary report on the proposal for an IAS machine by A.W. Burks, H.H. Goldstine and John von Neumann. June 1946

Within two years of these lectures the first stored program computer was operational, within 3 years there were 5 operational machines, and within 5 years stored program machines were commercially available. The Moore School Lectures, as they became known, were responsible for focusing all the leading developers of computing devices on a single problem:- How to design and build a stored program computer. It is interesting that despite being outnumbered and out-funded the British took, and held, the lead in this development effort between 1946 and 1953. In some areas such as business applications the British held the lead for much longer. How they were able to do this is not directly explained in any of the historical material available online, which tends to focus on individual development efforts and not on the larger picture.

Attendance at the Moore School Lectures

The lecturers who delivered the course are listed below.

Lecturer	Organization
Aiken, Howard H.	Harvard University
Burks, Arthur W.	Institute for Advanced Study, Princeton
Chu, J. Chuan	Moore School
Crawford, Perry U., Jr.	Office of Research and Inventions, U.S. Navy
Curtis, John H.	National Bureau of Standards
Eckert, J. Presper, Jr.	Electronic Control Company
Goldstine, Herman H.	Institute for Advanced Study, Princeton
Hartree, Douglas R.	University of Manchester
Lehmer, Derrick II.	University of California, Berkeley
Mauchley, John W.	Electronic Control Company
Moores, Calvin N.	Naval Ordnance Laboratory
Rademacher, Hans	University of Pennsylvania
Rajchman, Jan	RCA
Sharpless, T. Kite	Moore School
Sheppard,C. Bradford	Moore School
Stibitz, George	Independent consultant
Travis, Irven R.	Moore School
Von Neumann, John	Institute for Advanced Study, Princeton
Williams, Sam B.	Consultant, Moore School (Bell Telephone Laboratories)

40 lectures were delivered 5 days a week over 8 weeks. Most days a formal morning lecture lasting up to 3 hours was followed by an unstructured afternoon seminar.

The Lecture titles and the lecturer are listed below

	Lecturer	Lecture Title
1	George Stibitz	Introduction to the Course on Electronic Digital Computers
2	Irven Travis	The History of Computing Devices
3	J.W. Mauchly	Digital and Analog Computing Machines
4	D.H. Lehmer	Computing Machines for Pure Mathematics
5	D.R. Hartree	Some General Considerations in the Solutions of Problems in Applied Mathematics
6	H.H. Goldstine	Numerical Mathematical Methods I
7	H.H. Goldstine	Numerical Mathematical Methods II
8	A.W. Burks	Digital Machine Functions
9	J.W. Mauchly	The Use of Function Tables with Computing Machines
10	J.P. Eckert	A Preview of a Digital Computing Machine
11	C.B. Sheppard	Elements of a Complete Computing System
12	H.H. Goldstine	Numerical Mathematical Methods III
13	H.H. Aiken	The Automatic Sequence Controlled Calculator
14	H.H. Aiken	Electro-Mechanical Tables of the Elementary Functions
15	J.P. Eckert	Types of Circuit — General
16	T.K. Sharpless	Switching and Coupling Circuits
17	A.W. Burks	Numerical Mathematical Methods IV
18	H.H. Goldstine	Numerical Mathematical Methods V
19	Hans Rademacher	On the Accumulation of Errors in Numerical Integration on the ENIAC
20	J.P. Eckert	Reliability of Parts
21	C.B. Sheppard	Memory Devices
22	J.W. Mauchly	Sorting and Collating
23	J.P. Eckert C.B.	Sheppard Adders
24	J.P. Eckert	Multipliers
25	J.W. Mauchly	Conversions between Binary and Decimal Number Systems
26	H.H. Goldstine	Numerical Mathematical Methods VI
27	Chuan Chu	Magnetic Recording
28	J.P. Eckert	Tapetypers and Printing Mechanisms
29	J.H. Curtiss	A Review of Government Requirements and Activities in the Field of Automatic Digital Computing Machinery
30	H.H. Goldstine	Numerical Mathematical Methods VII
31	A.W. Burks	Numerical Mathematical Methods VIII
32	Perry Crawford	Application of Digital Computation Involving Continuous Input and Output Variables
33	J.P. Eckert	Continuous Variable Input and Output Devices
34	S.B. Williams	Reliability and Checking in Digital Computing Systems
35	J.P. Eckert	Reliability and Checking
36	C.B. Sheppard	Code and Control — I
37	J.W.Mauchly	Code and Control — II Machine Design and Instruction Codes
38	C.B. Sheppard	Code and Control — III
39	C.N. Mooers	Code and Control — IV Examples of a Three-Address Code and the Use of ‘Stop Order Tags’
40	John von Neumann	New Problems and Approaches
41	J.P. Eckert	Electrical Delay Lines
42	J.P. Eckert	A Parallel-Type EDVAC
43	Jan Rajchman	The Selectron
44	C.N. Mooers	Discussion of Ideas for the Naval Ordnance Laboratory Computing Machine
45	J.P. Eckert	A Parallel Channel Computing Machine
46	C.B. Sheppard	A Four-Channel Coded-Decimal Electrostatic Machine
47	T.K. Sharpless	Description of Serial Acoustic Binary EDVAC
48	J.W.Mauchly	Accumulation of Errors in Numerical Methods

The notes of the lectures published in The Moore School Lectures (Charles Babbage Institute Reprint) make the following observations about the lecturers.

Hartree was very forward looking and was excited by the mathematical potential of the stored program computer. On the other hand, Aiken was absorbed in his own way of doing things and does not appear to have been aware of the significance of the new electronic machines. The excellent review by John H. Curtis gave a very clear picture of contemporary computer development in the United States. But for most of the Students the real value was gained from the informal afternoon seminars.

Moore School Lecture “Students” are listed below. The term student is misleading as these people were the leading researchers in the field of computing.

Student	Organization
Alexander, Sam N.	National Bureau of Standards
Breiter, Mark	Office of the Chief of Ordnance, War Department
Brown, David R.	MIT Servomechanisms Laboratory
Cannon, Edward W.	National Bureau of Standards
Clark, Howard L.	General Electric Co.
Curtis, Roger	National Bureau of Standards
Elbourne, R. D.	Naval Ordnance Laboratory
Everett, Robert, R.	MIT Servomechanisms Laboratory
Galman, Herbert	Frankford Arsenal
Gard, Orin P.	Armament Laboratory, Wright Field
Gluck, Simon E.	Moore School
Gridley, D. H.	Naval Research Laboratory
Hobbs, G. W.	General Electric Co.
Horton, Arthur, B.	MIT
Loud, Warren S.	MIT
Lubkin, Samuel	Ballistics Research Laboratory, Aberdeen Proving Ground
Pendergrass, J. T.	OP-20G CNO Navy Department
Rees, David	Manchester University, England
Rosenbloom, Joshua	Frankford Arsenal
Sayre, Albert	Army Security Agency
Shaffer, Philip A., Jr.	Naval Ordnance Testing Station Pasadena, California
Shannon, Claude E.	Bell Telephone Laboratory
Smith, Albert E.	Navy Office of Research and Investigations
Suss, Louis	Naval Research Laboratory
Verzuh, Frank M.	Rockerfeller Electronic Computer Project, MIT
Wilkes, Maurice V.	Cambridge University
Wilson, Lou D.	MIT
Zagor, H. I.	Reeves Instrument Company

Additional Attendees included

Visitor	Organization
Jay Forrester	MIT
Robert Everett	MIT Servomechanisms Laboratory
David Brown	MIT Servomechanisms Laboratory

Other people attended but no record has been kept.

Analysis and Speculation

What follows is mostly speculation, I would be interested in evidence that supports or refutes these ideas.

I suspect the British were able to take the lead in computing in 1946 because the main challenge had become the rapid construction of a machine while solving the one remaining major technical problem – storing a program in memory. This problem was well understood in concept but the practical solution was more challenging than it appeared. As a result of their experience in the war the British were approximately 2 to 3 years in advance of the Americans in the crucial area of rapid prototyping and evolution of complex electronic devices. It was this ability that enabled them to take the lead from America.

During the War the British had developed RADAR further than any of the other combatants. This work occurred in secret at the Telecommunication Research Establishment TRE in Malvern. Meanwhile at Bletchley Park, they had secretly built and operated 10 Colossus Mk II code breaking machines. These machines were complex special purpose computing devices, they matched ENIAC in complexity and capability if not in size and generality. At the end of the war Britain had the largest concentration of electronic computing devices in the World and a significant number of engineers with practical skills in rapidly building complex electronics. The British centers of electrical engineering excellence which included; Bletchley Park, the Telecommunications Research Establishment (TRE) at Malvern, and The General Post Office Research Station at Dollis Hill, had all been driven by desperation to work with great speed and had each developed similar evolutionary prototyping approaches. The Colossus Mk I, and Mk II were constructed by Tommy Flowers in a matter of months at Dollis Hill, and the development of RADAR at the TRE had been similarly rapid. Americas leading center of excellence in the field of electronic computing – The Moore School – had become used to working at a slow pace. The ENIAC had taken 5 years to construct and was not completed until after the war ended.

In 1946 the Moore School’s leading experts left the school and went to other institutions. John von Neumann went with Herman Goldstine to the Institute for Advanced Study in Princeton and Mauchly and Eckert also left to setup their own company which was later purchased by Remington Rand. Both these groups lost valuable time in these reorganizations, however this was not the cause of the lead in computing passing to the UK. Similar reorganizations had happened already in the UK and other countries, as military programs were wound down and research expertise returned to civilian institutions.

By 1946 the conceptual architecture for a stored program computer was well understood by those interested in the field of electronic computing. Both John von Neumann and Alan Turing had developed and published designs. While these designs were revolutionary they were not particularly complex conceptually. As has been pointed out elsewhere a competent electrical engineer can grasp the main features of von Neumanns’ design in a day. Maurice Wilkes was famously given only one night to read the First Draft and decided there and then that this was the correct approach and that he would develop a machine along these lines – The EDSAC, generally accepted as the second operational stored program computer and the first machine to actually perform useful work. By the time the Moore School lectures had finished there were many people who understood exactly what needed to be done.

Two basic types of stored memory were under investigation, Serial Access Memory (SAM) and Random Access Memory (RAM). SAM could only be read in the order it was written while RAM was much faster as it could be read in any order. SAM devices in the form of mercury acoustic delay lines were already available as a result of RADAR development which need memory devices to improve image quality. RAM devices such as the Selectron were still under development but at the time of the Moore school lectures they were expected to be ready within a year.

In the UK all three centers of computer development hired people from either TRE Malvern, Bletchley Park or both to fill Senior positions. These men combined their expertise and rapidly developed plans for building stored program computers.

At Manchester University Max Neumann, who had directed efforts to break the Lorenz Cypher at Bletchley Park, became Fielden Professor of Pure Mathematics. He recruited, I.J. Good and D. Rees both from Bletchley. Meanwhile the Electrical Engineering Department recruited Freddie Williams from the TRE Malvern. Williams brought Tom Kilburn and later Geoff Tootill also from TRE to continue the development of a memory device based on the Cathode Ray Tubes CRT. Once at Manchester Williams and Kilburn rapidly perfected a working RAM based on the cathode ray tube. The Williams-Kilburn tube was working by March 1947 just over a year later The Baby, the first stored program computer was operational. The speed of this development must be compared with the ill-fated development of the Selectron which was running into difficulty in the US and was still not operational in the middle of 1948 when The Baby became operational. It was not used in a computer until the Johniac in 1953..

There is some dispute over who actually led the effort to build the Baby at Manchester the mathematics department or the Electrical Engineering departments. But what is clear is that men from TRE Malvern were able to solve the technical problems that were slowing efforts elsewhere and by employing a rapid evolutionary prototyping approach the Manchester University team was able to beat all the other teams in the UK and US to build a working stored program computer. The Baby ran its first successful program on 21 st June 1948.

At Cambridge University, Maurice Wilkes who had also been involved in Radar Development at TRE Malvern took a different approach. He chose to build a machine of modest capabilities from stock parts, or as near to stock as he could get – he chose mecury delay lines for the memory. The machine was called EDSACit was the second operational stored program computer after the Baby in Manchester and ran its first successful program on the 6 th May 1949. Wilkes was funded in part by the J. Lyons Company a forward thinking British teashop chain similar to today’s Starbucks. Lyons decided to build a computer of their own based on the EDSAC designed. Lyons hired John Pinkerton also of TRE who seconded two of his staff to Wilkes to help build EDSAC. In 1951 Loyns built their machine and called it LEO. It was the first business computer every used and was rapidly commercialized. IBMs first computer the Defense Calculator was not available until March 1953.

At the National Physics Laboratory (NPL) Alan Turing of Bletchley Park was leading efforts to build a computer of his own design called the Pilot ACE. Both Max Neumann and Alan Turing tried to recruit Tommy Flowers to help with development in 1946. Both failed, sadly he stayed at the Post Office. Alan Turing continued to use the Post Office research center at Dollis Hill to build mercury delay lines but Flowers and W. M. Combs another Bletchley man were being pulled onto other “more important” work and progress was delayed. Eventually Turing was persuaded to go to Manchester by Max Neuman. The Pilot ACE was constructed without him and was operational in 1951.

Meanwhile in America the situation was not improving. The Eckert Mauchly Computer Corporation built the BINAC for the Northrup Aircraft Corporation for a classified airborne application. It was tested and ran successfully for 44 hours in April 1949. It was then dismantled and delivered to Northrop in California were it was never successfully rebuilt. The IAS team led by von Neumann at Princeton was struggling with the Selectron and switched to the Williams-Kilburn tube soon after the Manchester team announced the Baby.

(The BINAC operation date (April 1949) needs to be confirmed. I found it in an Amazon review of the book ENIAC: The Triumphs and Tragedies of the World’s First Computer by Scott McCartney. The review was written on July 22 nd in 1999 by jbartik@ibm.net who claimed to have worked as a one of the first ENIAC programmers. If this date is correct it means BINAC as operational before EDSAC. )

In Australia yet another alumni of TRE Malvern was having more luck. Trevor Pearcey had moved from the UK to Australia in 1945 where he decided to design and build a computer. In 1948 he visited England and confirmed the soundness of his design. By November 1949 the CSIR Mk I was operational.

With 50+ year hindsight and knowledge of the secret British activities at TRE Malvern and Bletchley Park the Moore school lectures can be seen in a different light – one that raises many questions. To what extent did the hosts at the Moore School know about their British guests experience and skills? Had they known the truth would they have been so open with their information?. What would have happened if the British had been able to share their knowledge? One fact seems undeniable, only four stored program computers were operational before 1950 and three of these were built by people who had worked at TRE Malvern during the Second World War. The one exception, the BINAC, worked for 40 hours and was then dismantled never to work again. At the beginning of 1950 there were three working stored program computers in the world and none of them were in America. The expertise developed at Telecommunications Research Establishment (TRE) Malvern placed its engineers 2 to 3 years ahead of anyone else. How the British lost this lead is much less clear. The post war Austerity measures contributed and the failure of the UK government to make adequate investments may have been a factor. There was no direct equivalent to the US ARPA and the agencies that did exist were not well funded. Industry and universities failed to partner well in the way J. Lyons and Cambridge University had done. Whatever the case between 1950 and 1960 the British lost a 2 to 3 year lead in computing to America. But it may be fairer to say that the Americans took the lead back from the British.

Finally consider the case of David Rees of Manchester University. He must have had a uniquely interesting and frustrating experience at the Moore school in 1946. He had been sent by Max Neumann from Manchester University to the lectures as a “student” and yet he was the only person present who had first hand knowledge of Bletchley Park – the largest computing facility in the world, but, in the interests of British national security he was not allowed to talk about it.

Cyril Harris died 85 years ago today on 26th September 1918 in a German Prisoner of War Camp in France. He was 19 years old. As a child I met two of his brothers Arthur and Harry and can remember stories told at family gatherings about him and his siblings. I now own the few remaining documents that mark his brief existence. I have scanned these documents and present them here as a way of preserving them and his memory.

Cyril Harris was born in 1898 or 1899 at 16 Radcliffe Road, in the Hough (pronounced Hoff) district of Bolton, Lancashire in the United Kingdom. He was the seventh of eight children of William Henry Harris and his wife Mary Harris. His brothers were Robert, William, Harry, Jack, Arthur, and his sisters Annie, and Leah. He is said to have attended Hough School in Bolton, as did his brothers and sisters, from the age of 5 until he was 14 years old.

According to the Harris family, he was strongly advised to avoid involvement in the 1914-18 war by his brothers, all of them already serving in the forces and aware of the conditions in France and Belgium. Indeed there was no pressing need for him to do so and he was still under age for military service when he volunteered, which as probably in 1915 or 1916.

He joined the King’s Royal Rifle Corps and served in the 9th Battalion. He was captured in France and ended his days in a German prison camp. He died on the 26th September 1918 just a few weeks before the war ended. It is said that he died of starvation as, in the confusion of near defeat, his captors themselves had little food and still less for their prisoners.

His commemorative scroll and medals were sent to his brother Arthur when the war was ended and eventually, long after Arthur died, were found among the effects of their sister Leah. He is buried in the military cemetery at Glageon near Avenses in northern France some 10-15 km from Arras. In the 1960s his brother Arthur visited the cemetery and returned with a few photographs of the grave.

A Letter to me from Peter. M. Harris my father (the grandson of Cyril’s older brother Robert) 9th September 2000

This article appeared in the Bolton Evening News, 1918 (Probably October or November). It is not strictly accurate as Cyril’s brother Jack was in the navy and therefore not a soldier. This is the only existing photo of Cyril.

Six or seven months after the article in the Bolton evening news was published his mother received this form letter from the War Office

No 14 5220 (If replying, please quote above No.) Army from B 104-82

Kings Royal Rifle Corps Record Office, Winchester May 12th 1919

Madam

It is my painful duty to inform you that a report has been received from the War Office notifying the death of :- (No.) R/9237 (Rank) Rfm (Name) C. Harris (Regiment) 9th Kings Royal Rifle Corps. which occurred at Trelon. on the 26th September 1918. The report is to the effect that he Died whilst a Prisoner of war (Cause not stated), I am to express the regret of the Army Council at the soldier’s death In his Country’s services.

I am to add that any information that may be received as to the soldier’s burial will be communicated to you in due course. A separate leaflet dealing more fully with this subject is enclosed.

Buried in Glageon Cemetery Grave No 172 H

I am, Madam Your obedient Servant, F. Sco? for L’Col Officer in Charge of Records

His grave (Reference No. II. H. 6.) is in the Northern extension of the Glageon Communal Cemetery, France. and is maintained by the Commonwealth War Graves Commission who also maintain his Online Registry entry

This picture shows Cyril Harris’s brother Arthur Harris standing next to his grave on May 5 th 1960. The date is carefully recorded on the back of the photo and seems to be significant since Cyril and Arthur’s elder brother William Harris. Private 11511. 2nd Bn., Duke of Wellington’s (West Riding Regt.) died on May 5th 1915 at Hill 60 during the second Battle of Ypres. It seems Arthur timed his visit to France to coincide with the 45 anniversary of William’s death.

Finally Cyril must have nominated his brother Arthur as next of kin when he joined the army since his medal and commemorative scroll were sent to Arthur after the war.

Update 2011-01. Since writing this post another photograph of Cyril has been discovered. This is a much clearer photograph that really highlights Cyril’s youth

RMS (Risk Management Solutions) is a small US company that specializes in catastrophe models for the insurance industry. These models cover natural perils such as earthquakes, hurricanes, and other windstorms. In 2002 RMS produced a report entitled Accessing Workers Comp Risk from Earthquakes (updated version here). What if the 1906 Great San Francisco Earthquake occurred today. The point of this report was to draw the attention of catastrophe risk managers in the insurance industry to the potentially high costs of workers compensation in large catastrophes. It also makes fairly sobering reading for people who work in San Francisco.

RMS assumed the replay of the Great Quake would occur at peak office occupancy hours; mid-afternoon, mid-week. The Diagram below shows the relative ground shaking used to calculate potential losses

The following table shows the potential losses in workers compensation from a repeat of the 1906 earthquake compared to equivalent losses from the World Trade Center Attack.

Workers Compensation	1906 San Francisco Earthquake Repeat		World Trade Center Attack
	Expected	90% Confidence
Injuries	37,000	78,000	Unknown
Deaths	3,000	5,000	2,700
Insured Loss	$3.4B	$7.1B	$2.5B – $5.0B